Ideal Gas Law Applicability: Does The Center Of Jupiter Under Go Ideal Gas Law
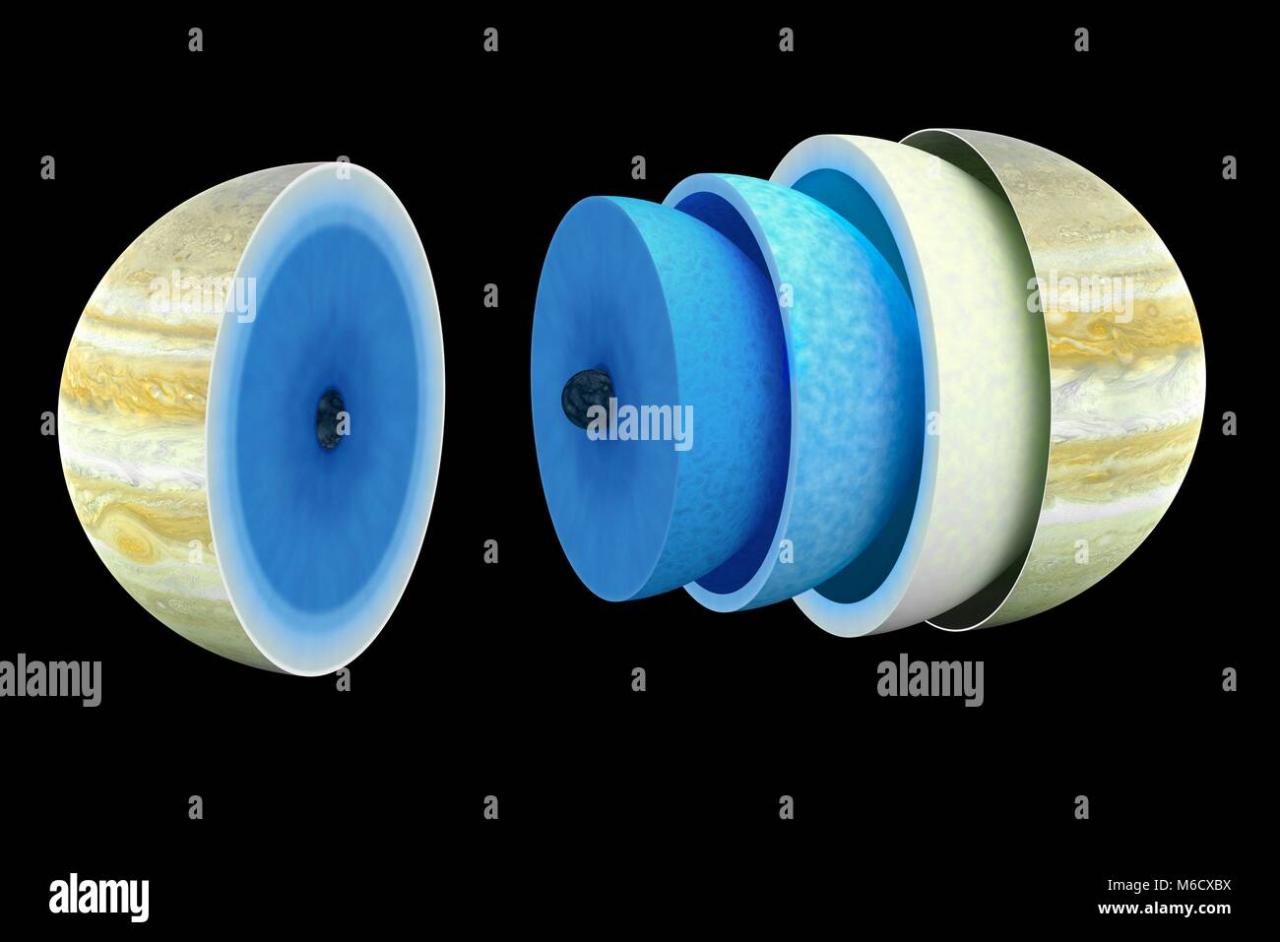
The ideal gas law, PV = nRT, provides a simplified model for the behavior of gases. While incredibly useful for many applications, its accuracy depends heavily on the conditions under which the gas exists. Understanding these conditions, and the limitations of the model, is crucial for accurately describing gas behavior, especially in extreme environments like Jupiter’s core.
The ideal gas law assumes that gas particles have negligible volume and exert no intermolecular forces on each other. These assumptions hold true for many gases under normal temperatures and pressures. However, at high pressures and densities, these assumptions break down, leading to significant deviations from ideal behavior.
Limitations of the Ideal Gas Law at High Pressures and Densities, Does the center of jupiter under go ideal gas law
At high pressures, gas molecules are forced closer together. Their own volume becomes a significant fraction of the total volume, contradicting the ideal gas assumption of negligible molecular volume. Simultaneously, at high densities, intermolecular forces, both attractive and repulsive, become increasingly important. These forces significantly influence the gas’s pressure and volume, causing deviations from the ideal gas law’s predictions. The increased interaction between molecules leads to a reduction in the effective volume available for the gas to occupy and influences the overall pressure. For example, at extremely high pressures, such as those found deep within gas giants like Jupiter, the behavior of hydrogen and helium deviates substantially from the ideal gas law. Compressibility factors, which quantify the deviation from ideality, become significantly larger than 1 under these conditions.
Comparison of Hydrogen and Helium Under Extreme Pressure and Temperature
Both hydrogen and helium, the primary constituents of Jupiter’s atmosphere, exhibit non-ideal behavior under extreme pressure and temperature. However, their behavior differs slightly due to variations in their intermolecular forces. Helium, being a noble gas with very weak intermolecular forces, shows a slightly closer adherence to the ideal gas law at moderate deviations from standard conditions compared to hydrogen. Hydrogen, on the other hand, experiences stronger intermolecular forces at high densities due to its potential for weak van der Waals interactions, leading to more significant deviations from ideal behavior than helium under similar conditions. At the pressures and temperatures found in Jupiter’s core, both gases exist in a metallic state, dramatically altering their properties and rendering the ideal gas law entirely inapplicable. The metallic state arises due to the extreme compression, forcing electrons to become delocalized and behave as a fluid, significantly impacting the overall behavior of the system.
Real Gases and Their Deviation from Ideal Behavior
A real gas is a gas that does not adhere perfectly to the ideal gas law. The deviations arise from the fact that real gas molecules do possess volume and do interact with each other through attractive and repulsive forces. These forces and molecular volume become increasingly significant as pressure increases and temperature decreases, causing the real gas to deviate from the predictions of the ideal gas law. Various equations of state, such as the van der Waals equation, attempt to account for these deviations by introducing correction factors to the ideal gas law. These equations incorporate parameters that represent the volume of the gas molecules and the strength of intermolecular forces, providing a more accurate description of real gas behavior under non-ideal conditions. For instance, the van der Waals equation,
(P + a(n/V)²)(V – nb) = nRT
, incorporates the constants ‘a’ and ‘b’ to account for intermolecular attractive forces and molecular volume respectively.
Alternative Models for Jupiter’s Interior
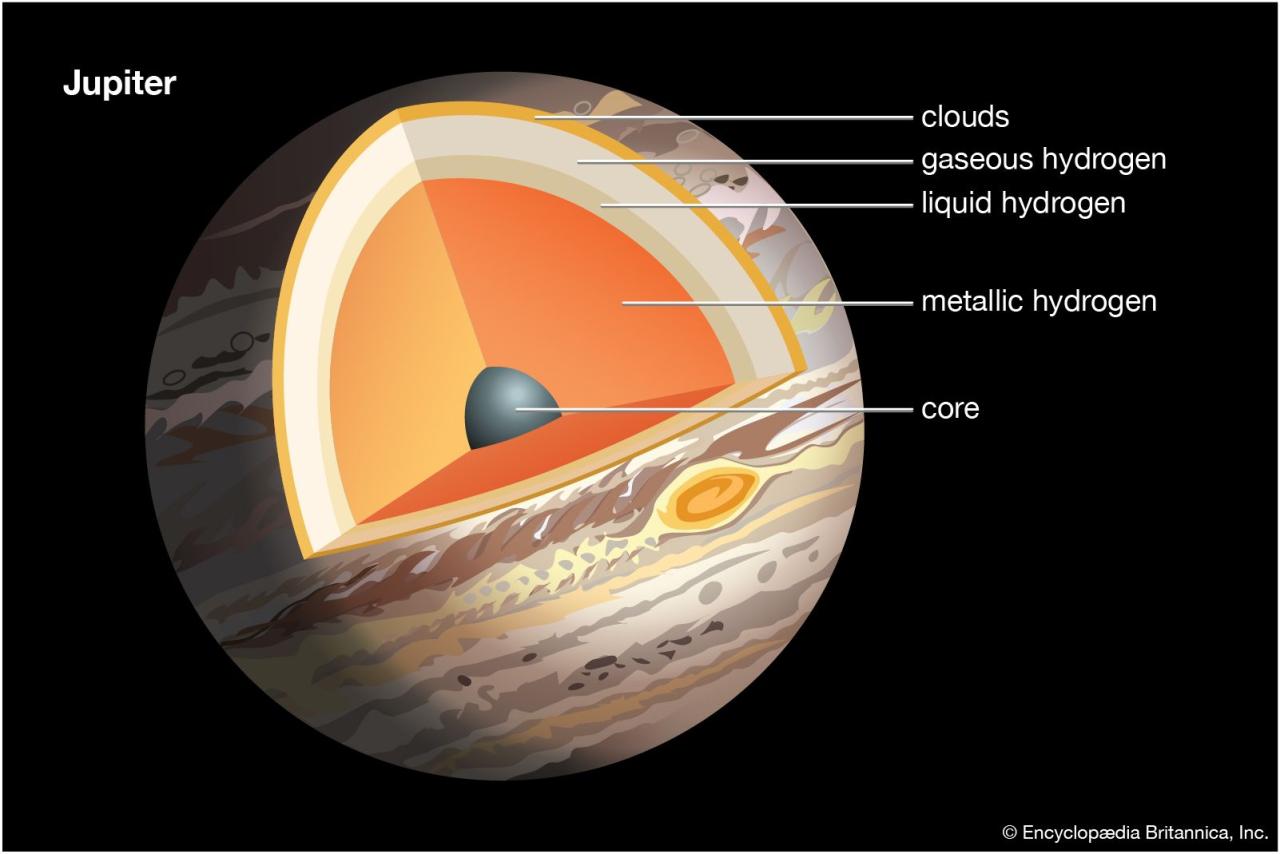
While the ideal gas law provides a simplified understanding of Jupiter’s atmosphere, it falls short in accurately describing the behavior of matter under the extreme pressure and temperature conditions found deeper within the planet. More sophisticated models are necessary to account for the complex interactions of hydrogen and helium at these extreme conditions. These models incorporate quantum mechanical effects and consider the transition of hydrogen from a gaseous to a metallic state.
The limitations of the ideal gas law become particularly apparent when considering the immense pressure at Jupiter’s core, where pressures are millions of times that of Earth’s atmosphere. At these pressures, the assumptions of negligible intermolecular forces and negligible molecular volume, central to the ideal gas law, are no longer valid. Therefore, alternative models that account for these interactions are crucial for a more accurate representation of Jupiter’s internal structure.
Equation of State Models
Equation of state (EOS) models are fundamental to understanding Jupiter’s interior. These models mathematically relate pressure, temperature, and density of matter under various conditions. Unlike the ideal gas law, which assumes simple relationships, EOS models incorporate complex interactions between particles, including those based on quantum mechanics. They consider factors such as electron degeneracy pressure, which becomes significant at high densities, and the phase transitions of hydrogen from a molecular gas to a metallic liquid. Different EOS models utilize varying levels of approximation and complexity, reflecting different assumptions about the composition and interactions within Jupiter’s interior. For instance, some models may employ sophisticated numerical techniques to solve complex quantum mechanical problems, while others might use simpler, analytical approximations. The accuracy of these models is often tested against observational data such as Jupiter’s gravitational field and its emitted heat.
Metallic Hydrogen Models
A significant aspect of many models is the consideration of metallic hydrogen. Under the extreme pressures within Jupiter’s interior, molecular hydrogen (H2) is predicted to undergo a phase transition, becoming metallic hydrogen. This transition significantly alters the electrical and thermal conductivity of the material. Metallic hydrogen models incorporate this phase transition, influencing calculations of the planet’s internal temperature profile and magnetic field generation. The exact pressure at which this transition occurs is still debated, with predictions varying depending on the specific EOS model used. The properties of metallic hydrogen remain largely theoretical, as it hasn’t been created and studied in a laboratory setting under the necessary pressures. However, experimental work under high-pressure conditions provides insights into the potential behavior of metallic hydrogen, informing the development of these models. The presence of metallic hydrogen is considered crucial for explaining Jupiter’s powerful magnetic field.
Multi-Component Models
Jupiter’s composition is not solely hydrogen and helium. Trace amounts of other elements and compounds are present, which can influence the overall equation of state. Multi-component models account for these minor constituents, considering their impact on pressure, temperature, and density profiles. These models are particularly important when attempting to understand the distribution of heavier elements within Jupiter, providing insights into the planet’s formation and evolution. The inclusion of these additional components increases the complexity of the calculations but offers a more realistic representation of Jupiter’s internal structure. For example, the presence of heavier elements in the core could affect the overall density profile, influencing predictions of the planet’s moment of inertia.

Tim Redaksi