Ideal Gas Law in Compressible Flow Calculations: Is The Ideal Gas Law Used For Aerodynamics
The ideal gas law, while a simplification of real gas behavior, plays a crucial role in understanding and modeling compressible flows. Its simplicity allows for the derivation of fundamental equations and provides a reasonable approximation for many aerodynamic applications, especially at lower altitudes and moderate speeds. Deviations from ideal gas behavior become more significant at high altitudes or extreme conditions, necessitating the use of more complex equations of state.
The Ideal Gas Law’s Role in Deriving Compressible Flow Equations
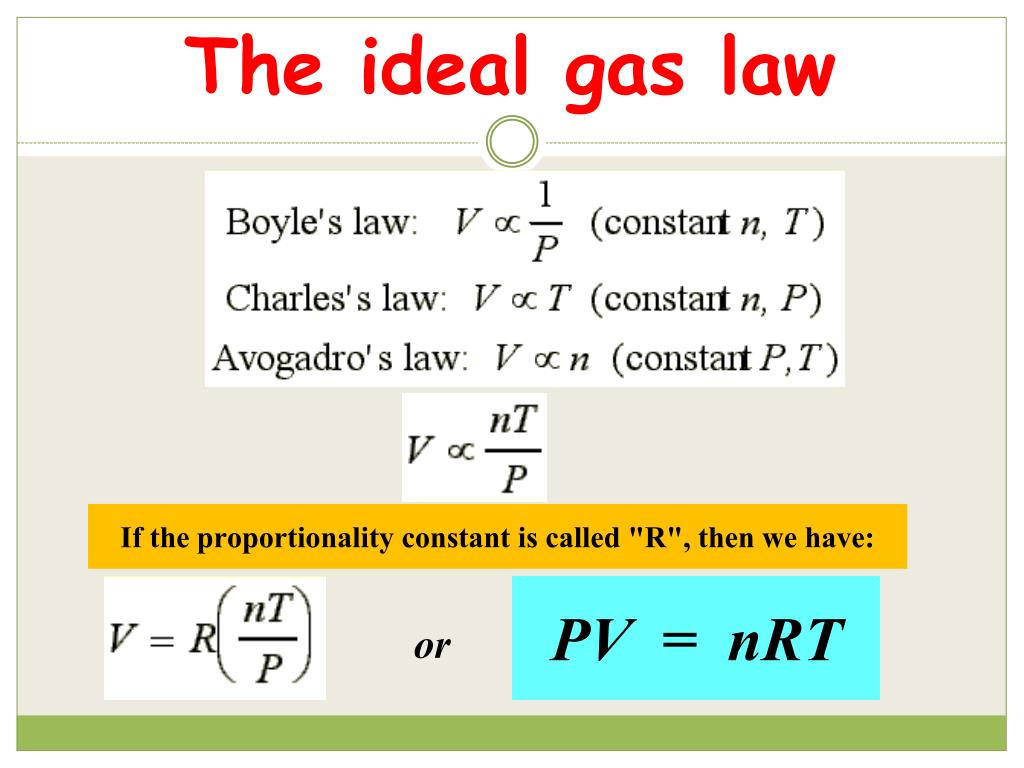
The ideal gas law, expressed as
PV = mRT
where P is pressure, V is volume, m is mass, R is the specific gas constant, and T is temperature, is fundamental to deriving other equations governing compressible flow. For instance, the continuity equation, which describes the conservation of mass in a fluid flow, utilizes density (ρ), which is directly related to pressure and temperature through the ideal gas law (ρ = P/(RT)). Similarly, the energy equation, describing the conservation of energy, incorporates the ideal gas law to relate changes in internal energy to changes in temperature. These equations are then solved simultaneously to analyze and predict compressible flow behavior.
Determining the Speed of Sound Using the Ideal Gas Law
The speed of sound (a) in a gas is directly related to its thermodynamic properties. The ideal gas law facilitates the derivation of the following equation for the speed of sound:
a = √(γRT)
where γ is the ratio of specific heats (Cp/Cv) for the gas. This equation demonstrates the direct dependence of the speed of sound on temperature and the gas constant, highlighting the importance of the ideal gas law in understanding acoustic phenomena within compressible flows.
Calculating Air Density at a Given Altitude and Temperature
A step-by-step procedure for calculating air density (ρ) at a given altitude and temperature using the ideal gas law is as follows:
1. Obtain the necessary data: Determine the pressure (P) and temperature (T) at the specified altitude. Standard atmospheric models, such as the International Standard Atmosphere (ISA), provide this information.
2. Determine the specific gas constant: For dry air, the specific gas constant (R) is approximately 287 J/(kg·K).
3. Apply the ideal gas law: Use the equation
ρ = P/(RT)
to calculate the density. Ensure consistent units throughout the calculation (e.g., Pascals for pressure, Kelvin for temperature).
4. Interpret the result: The calculated density represents the air density at the specified altitude and temperature.
For example, at an altitude where the pressure is 50,000 Pa and the temperature is 250 K, the air density would be approximately 0.7 kg/m³.
Ideal Gas Law vs. More Complex Equations of State, Is the ideal gas law used for aerodynamics
For many aerodynamic applications, the ideal gas law provides a sufficiently accurate representation of air behavior. However, at high pressures and low temperatures, deviations from ideal gas behavior become more pronounced. Consider a high-speed flight scenario at high altitude. The ideal gas law might underestimate the density at extremely low temperatures and relatively high pressures experienced near the aircraft’s leading edge. More complex equations of state, such as the van der Waals equation or real gas models accounting for intermolecular forces, would then yield a more accurate prediction of density and other thermodynamic properties. The difference between results from the ideal gas law and a more complex equation of state will depend on the specific conditions and the level of accuracy required.
Effect of Temperature and Pressure on Air Density
The following table illustrates the effect of varying temperature and pressure on air density, calculated using the ideal gas law:
| Pressure (Pa) | Temperature (K) | Density (kg/m³) | Example Scenario |
|---|---|---|---|
| 101325 | 288.15 | 1.225 | Sea level, standard atmospheric conditions |
| 50000 | 250 | 0.700 | High altitude, cold conditions |
| 100000 | 300 | 1.162 | Higher pressure, warmer conditions |
| 20000 | 220 | 0.407 | Very high altitude, very cold |

Tim Redaksi