Illustrative Examples: What Would A Graph Of Charles Law Look Like
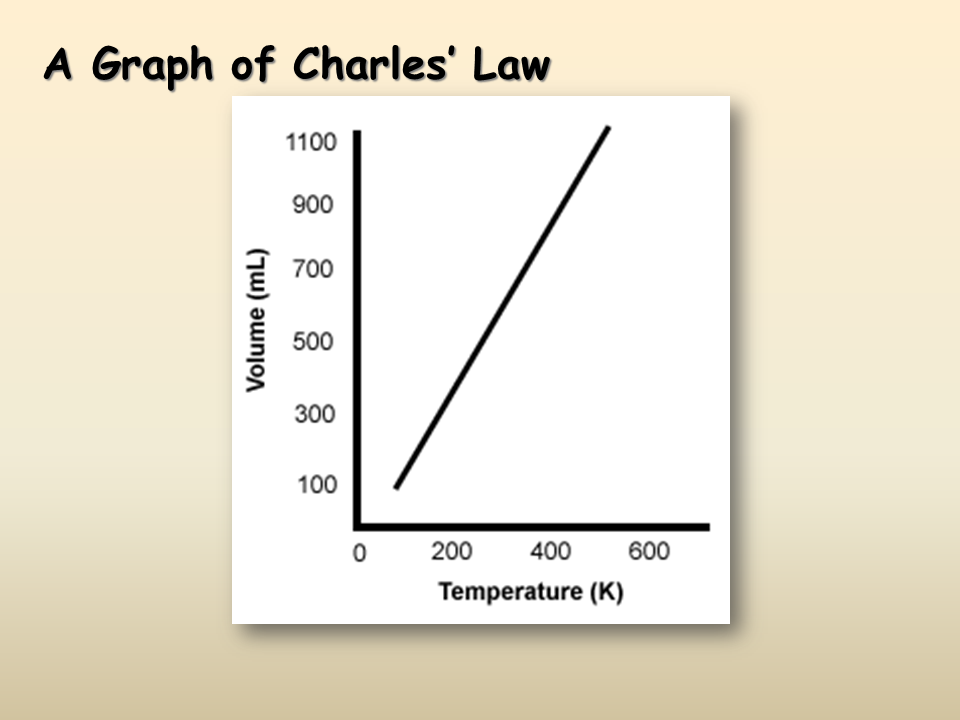
Charles’s Law, which states that the volume of a gas is directly proportional to its absolute temperature at constant pressure, can be elegantly demonstrated through a simple experiment. Understanding this relationship is crucial in various fields, from meteorology to engineering. The following example details a hypothetical experiment and its graphical representation.
Hypothetical Experiment Demonstrating Charles’s Law
A sealed, flexible container (like a balloon) is filled with a known volume of air at room temperature. The initial volume (V₁) is measured to be 200 mL at an initial temperature (T₁) of 20°C (293.15 K). The apparatus consists of the balloon, a thermometer accurate to 0.1°C, and a graduated cylinder for precise volume measurement. The balloon is then submerged in a series of water baths maintained at different temperatures, ensuring constant atmospheric pressure. At each temperature, the new volume (V₂) of the balloon is carefully measured. The experiment is repeated for temperatures of 40°C (313.15 K), 60°C (333.15 K), 80°C (353.15 K), and 100°C (373.15 K). The following data is obtained:
| Temperature (°C) | Temperature (K) | Volume (mL) |
|---|---|---|
| 20 | 293.15 | 200 |
| 40 | 313.15 | 214 |
| 60 | 333.15 | 228 |
| 80 | 353.15 | 242 |
| 100 | 373.15 | 256 |
Graph Construction from Experimental Data
To construct a graph illustrating Charles’s Law, the absolute temperature (in Kelvin) is plotted on the x-axis and the volume (in mL) is plotted on the y-axis. Each data point from the experiment is carefully plotted on the graph. For example, the first data point (293.15 K, 200 mL) would be plotted at the intersection of the 293.15 K mark on the x-axis and the 200 mL mark on the y-axis. This process is repeated for all five data points. Once all points are plotted, a line of best fit is drawn through the points. This line should be as close as possible to all the points, with approximately an equal number of points above and below the line. The line should not necessarily pass through every single point, as experimental error is inherent. The line should extend to the origin (0,0), reflecting the theoretical point where volume would be zero at absolute zero temperature (-273.15°C or 0 K). Note that this is a theoretical extrapolation and is not physically attainable.
Graphical Representation of Charles’s Law, What would a graph of charles law look like
The graph would show a straight line passing through the origin (0,0), with a positive slope. The x-axis is labeled “Absolute Temperature (K)” and the y-axis is labeled “Volume (mL)”. The title of the graph could be “Charles’s Law: Volume vs. Temperature”. Each data point from the table above would be clearly visible on the graph. The line of best fit demonstrates the direct proportionality between volume and absolute temperature. The slope of the line represents the constant of proportionality (k) in the equation
V = kT
, where V is volume and T is absolute temperature. A caption below the graph might read: “This graph illustrates Charles’s Law, showing the direct proportionality between the volume of a gas and its absolute temperature at constant pressure. The straight line through the origin represents this relationship, with the slope representing the proportionality constant.”

Tim Redaksi