Introduction to Charles’s Law: What Are The Variables Of Charles Law
Charles’s Law is a fundamental gas law that describes the relationship between the volume and temperature of a gas when the pressure and the amount of gas are held constant. It’s a cornerstone of our understanding of how gases behave under varying conditions and has significant implications in various scientific and engineering fields.
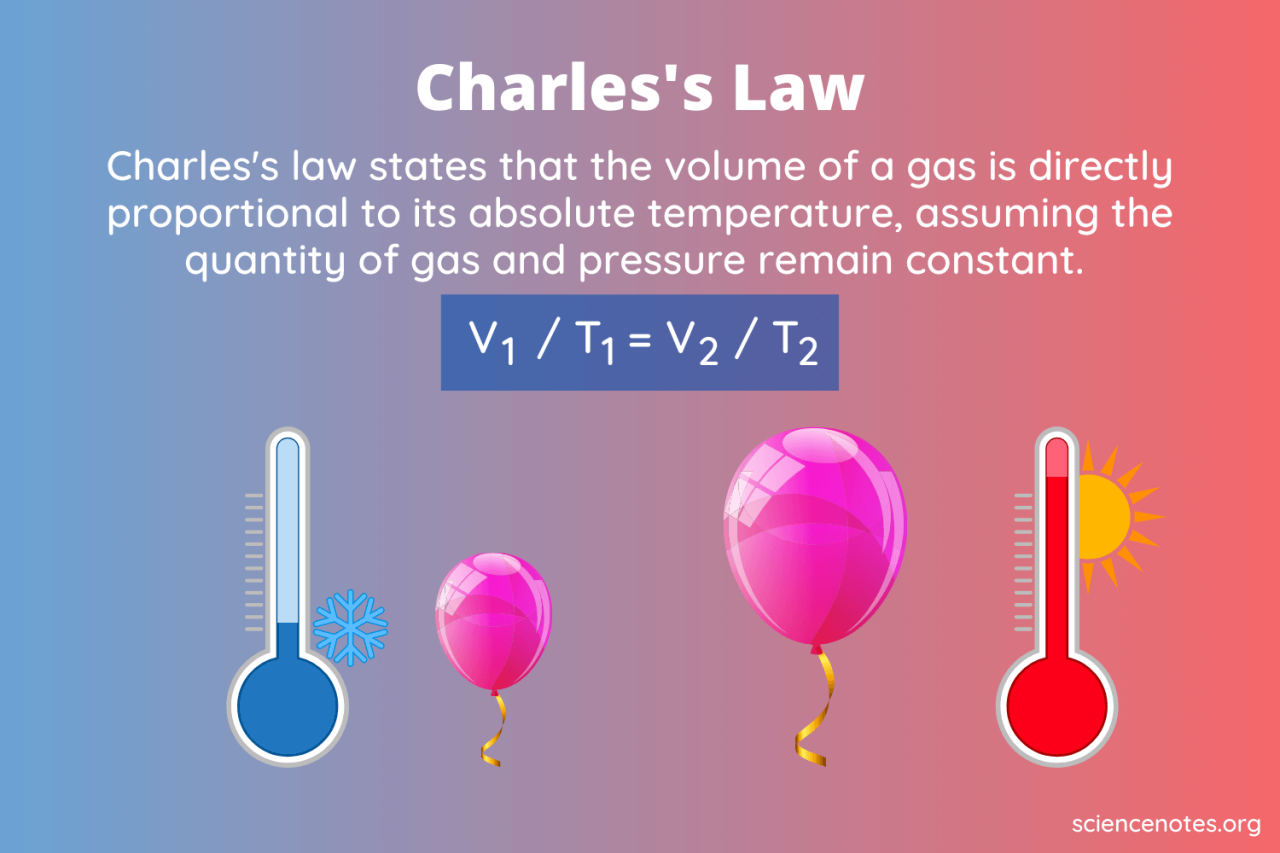
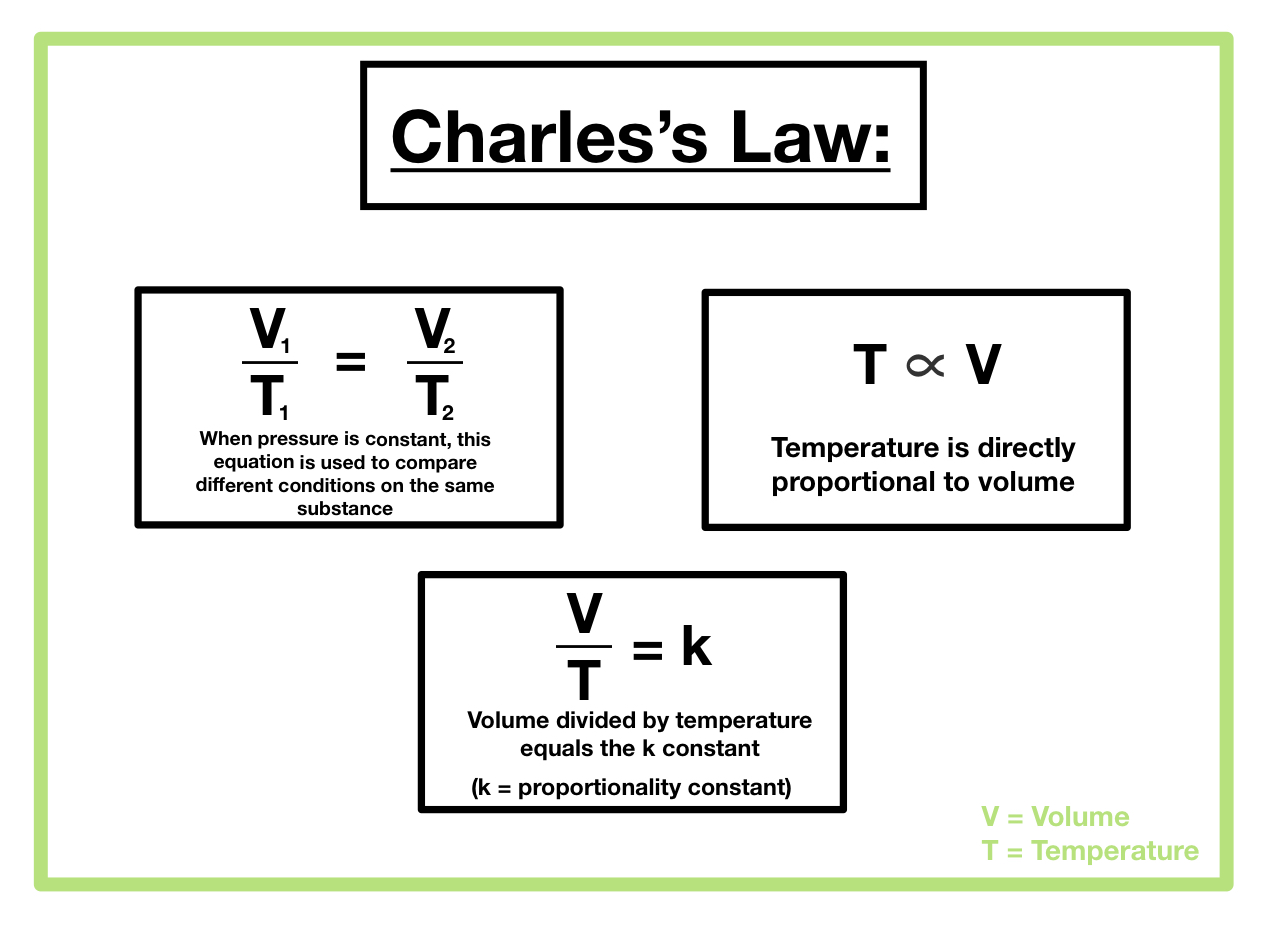
Charles’s Law states that the volume of a given amount of gas is directly proportional to its absolute temperature, provided the pressure remains constant. In simpler terms, as the temperature of a gas increases, its volume increases proportionally, and vice versa. This relationship can be expressed mathematically as V/T = k, where V is the volume, T is the absolute temperature (in Kelvin), and k is a constant.
Historical Overview of Charles’s Law
While the law is named after Jacques Charles, a French physicist, his work wasn’t the first to explore this relationship. Early experiments by others hinted at the connection between gas volume and temperature. However, Charles’s meticulous experiments in the late 18th century provided strong experimental evidence supporting the direct proportionality. He observed that various gases expanded by the same fraction when heated through the same temperature interval, under constant pressure. Although Charles did not publish his findings, Joseph Louis Gay-Lussac later independently confirmed and published the law in 1802, acknowledging Charles’s prior work. Gay-Lussac’s experiments involved more extensive data, solidifying the law’s acceptance within the scientific community. This demonstrates the collaborative nature of scientific discovery, with one researcher’s work building upon and validating the findings of others. The precise mathematical formulation, V₁/T₁ = V₂/T₂, which is commonly used today to solve problems related to Charles’s Law, is a direct consequence of this principle of direct proportionality. This equation allows us to predict the change in volume of a gas given a change in temperature, or vice versa, under constant pressure conditions.
Identifying the Variables in Charles’s Law

Charles’s Law describes the relationship between the volume and temperature of a gas when the pressure is held constant. Understanding the variables involved is crucial for applying this law correctly in various scientific and engineering contexts. This section will clearly define the dependent and independent variables, and explain the significance of constant pressure.
What are the variables of charles law – Charles’s Law, in its simplest form, states that the volume of a gas is directly proportional to its absolute temperature, provided the pressure remains constant. This means that as the temperature increases, the volume of the gas also increases, and vice versa. This relationship can be expressed mathematically as V/T = k, where V represents volume, T represents absolute temperature (in Kelvin), and k is a constant.
The Dependent Variable in Charles’s Law
The dependent variable in Charles’s Law is the volume (V) of the gas. This is because the volume is observed to change in response to changes in the independent variable (temperature). The volume is directly affected by the temperature; if the temperature increases, the volume increases proportionally, and if the temperature decreases, the volume decreases proportionally. Experimental measurements of volume are taken to observe this dependence on temperature.
The Independent Variable in Charles’s Law
The independent variable in Charles’s Law is the absolute temperature (T) of the gas. This is the variable that is manipulated or controlled during an experiment to observe its effect on the dependent variable (volume). The experimenter chooses specific temperatures, and the corresponding volumes are then measured. The temperature is the driving force behind the observed changes in volume, making it the independent variable.
The Role of Constant Pressure in Charles’s Law
Constant pressure is a crucial condition for Charles’s Law to hold true. If the pressure is allowed to change, the relationship between volume and temperature becomes more complex and is no longer accurately described by the simple equation V/T = k. Maintaining constant pressure ensures that the only factor influencing the volume is the temperature. This controlled environment allows for a clear observation of the direct proportionality between volume and temperature. Changes in pressure would introduce additional variables that would confound the results and obscure the direct relationship between volume and temperature.
| Variable | Description |
|---|---|
| Volume (V) | The dependent variable; the volume of the gas changes in response to changes in temperature. It is typically measured in liters (L) or cubic meters (m³). |
| Absolute Temperature (T) | The independent variable; the temperature of the gas (measured in Kelvin) is manipulated to observe its effect on the volume. |
| Pressure (P) | This variable is held constant throughout the experiment. Its constancy is crucial for the validity of Charles’s Law. |
Limitations and Assumptions of Charles’s Law
Charles’s Law, while a useful simplification of gas behavior, is not universally applicable. Its accuracy depends on several assumptions about the gas and its environment, and deviations from these assumptions lead to limitations in its predictive power. Understanding these limitations and underlying assumptions is crucial for correctly applying the law.
The primary limitation stems from the ideal gas assumption. Charles’s Law, in its simplest form, assumes that the gas behaves ideally, meaning there are no intermolecular forces between gas particles and that the volume of the gas particles themselves is negligible compared to the total volume of the gas. Real gases, however, exhibit intermolecular forces (attractive and repulsive) and possess finite molecular volumes. These deviations become more significant at higher pressures and lower temperatures, where the intermolecular forces become more influential. At extremely low temperatures, gases can even liquefy, completely invalidating the assumptions of Charles’s Law.
Assumptions Made When Applying Charles’s Law, What are the variables of charles law
Charles’s Law is based on several crucial assumptions. These assumptions, while simplifying the model, limit its applicability to real-world scenarios. Accurate application requires a careful consideration of the conditions under which these assumptions hold true.
The primary assumptions include a constant amount of gas (number of moles), a constant pressure, and that the gas behaves ideally (negligible intermolecular forces and negligible particle volume). If any of these conditions are not met, the relationship between volume and temperature predicted by Charles’s Law will not accurately reflect the gas’s behavior. For instance, if the pressure changes significantly during the heating or cooling process, the volume change will not be solely determined by the temperature change.
Comparison of Charles’s Law with Other Gas Laws
Several gas laws describe the behavior of gases under different conditions. Comparing these laws highlights their individual scopes and limitations. The following table summarizes key differences:
| Gas Law | Equation | Variables | Assumptions |
|---|---|---|---|
| Charles’s Law | V1/T1 = V2/T2 | Volume (V), Temperature (T) (constant pressure and amount of gas) | Constant pressure, constant amount of gas, ideal gas behavior |
| Boyle’s Law | P1V1 = P2V2 | Pressure (P), Volume (V) (constant temperature and amount of gas) | Constant temperature, constant amount of gas, ideal gas behavior |
| Gay-Lussac’s Law | P1/T1 = P2/T2 | Pressure (P), Temperature (T) (constant volume and amount of gas) | Constant volume, constant amount of gas, ideal gas behavior |
| Ideal Gas Law | PV = nRT | Pressure (P), Volume (V), Temperature (T), Amount of gas (n) | Ideal gas behavior (negligible intermolecular forces and particle volume) |
Real-World Applications of Charles’s Law
Charles’s Law, describing the direct relationship between the volume and temperature of a gas at constant pressure, finds numerous practical applications in various fields. Understanding this relationship is crucial for predicting and controlling gas behavior in diverse real-world scenarios. Its implications range from everyday occurrences to sophisticated technological processes.
The significance of Charles’s Law lies in its ability to predict the changes in gas volume due to temperature fluctuations. This predictive power is essential for designing and operating systems where gases are involved, ensuring safety and efficiency.
Examples of Charles’s Law in Action
Several everyday phenomena and industrial processes rely heavily on the principles of Charles’s Law. The following examples highlight its practical importance.
- Hot Air Balloons: The inflation of hot air balloons is a classic demonstration of Charles’s Law. Heating the air inside the balloon causes its volume to expand, making it less dense than the surrounding cooler air, resulting in buoyancy and lift. The pilot controls the altitude by adjusting the temperature of the air within the balloon.
- Weather Forecasting: Meteorologists use Charles’s Law to understand and predict weather patterns. Changes in air temperature directly affect air volume, influencing atmospheric pressure and wind currents. Accurate weather forecasting relies on precisely modeling these temperature-volume relationships.
- Tire Pressure: On a hot summer day, the air inside car tires expands due to the increased temperature. This expansion can lead to higher tire pressure, potentially causing a blowout if not monitored. Conversely, in cold weather, tire pressure decreases, affecting vehicle handling and fuel efficiency. Understanding this temperature-pressure relationship, fundamentally based on Charles’s Law, is essential for safe driving.
- Aerospace Engineering: The design of aircraft and spacecraft involves careful consideration of Charles’s Law. Changes in atmospheric temperature at different altitudes affect the volume of gases within the aircraft, influencing its performance and stability. Precise calculations are necessary to ensure safe and efficient operation at varying altitudes and temperatures.
- Industrial Processes: Many industrial processes involve gases at high temperatures and pressures. Understanding Charles’s Law is critical for designing and operating equipment such as gas pipelines, storage tanks, and chemical reactors. Accurate predictions of gas volume changes are crucial for safety and process optimization.
Crucial Instances Requiring Charles’s Law Understanding
In several situations, a thorough understanding of Charles’s Law is not just beneficial but absolutely crucial for safety and operational efficiency.
- Scuba Diving: Changes in water temperature affect the volume of air in scuba tanks. Divers must account for these changes to avoid running out of air prematurely or experiencing dangerous pressure imbalances. Ignoring Charles’s Law in this context can have life-threatening consequences.
- Refrigeration Systems: Refrigeration systems rely on the expansion and compression of gases to transfer heat. Charles’s Law plays a critical role in understanding the efficiency and performance of these systems. Improper design or operation can lead to inefficiencies and equipment malfunction.
- High-Altitude Flying: At high altitudes, the atmospheric pressure is significantly lower, and the temperature is much colder. Understanding Charles’s Law is vital for pilots to ensure the safe and efficient operation of aircraft engines and other systems under these extreme conditions.
Experimental Verification of Charles’s Law
Charles’s Law, stating that the volume of a gas is directly proportional to its absolute temperature at constant pressure, can be experimentally verified using relatively simple apparatus. This allows for a hands-on understanding of the relationship between temperature and volume in gases. The following experiment Artikels a straightforward method for demonstrating this fundamental gas law.
Experimental Procedure
A simple experiment to verify Charles’s Law involves heating a fixed mass of gas in a sealed container and observing the change in its volume. Accurate measurements of temperature and volume are crucial for obtaining reliable results. Precise control of pressure is also essential to ensure the validity of the experiment.
- Materials: A sealed glass flask or syringe (graduated to allow volume measurement), a thermometer capable of measuring a temperature range encompassing both the initial and final temperatures, a heat source (e.g., a hot water bath or Bunsen burner with a heat-resistant mat), a clamp stand and boss head to securely hold the flask/syringe, and a ruler or other measuring device for precise volume readings. For greater accuracy, a pressure sensor can also be incorporated. Safety glasses are mandatory.
- Initial Setup: Securely clamp the flask or syringe to the stand. Record the initial volume (V1) of the gas at room temperature. Record the initial temperature (T1) using the thermometer. Ensure the pressure remains constant throughout the experiment. This might involve conducting the experiment in an environment with stable atmospheric pressure, or using a pressure-controlled system.
- Heating and Measurement: Gradually heat the flask/syringe using the hot water bath or Bunsen burner, ensuring even heating to prevent localized temperature variations. Continuously monitor the temperature and record the volume (V2) at several different temperatures (T2, T3, etc.). Allow sufficient time for the gas to reach thermal equilibrium at each temperature before taking measurements. Remember to record temperature in Kelvin (K) by adding 273.15 to the Celsius (°C) reading.
- Data Analysis: Plot the volume (V) against the absolute temperature (T) on a graph. If Charles’s Law holds true, the resulting graph should be a straight line passing through the origin (0,0), demonstrating a direct proportionality between volume and absolute temperature. Any deviation from a straight line may indicate experimental error or limitations of Charles’s Law under the specific conditions of the experiment.
Data Representation and Analysis
The collected data should be tabulated, showing corresponding values of volume and temperature. A graph plotting volume (V) on the y-axis against absolute temperature (T) on the x-axis is crucial for visualizing the relationship. The slope of the line of best fit through the data points represents the proportionality constant for the specific gas sample used in the experiment. The closer the data points fall to a straight line passing through the origin, the better the experimental verification of Charles’s Law.

Tim Redaksi